Pentagon abcde is rotated 90 degrees counterclockwise – As we delve into the fascinating world of geometry, let us embark on a journey to explore the intricate transformations that unfold when Pentagon ABCDE undergoes a 90-degree counterclockwise rotation. This exploration promises to unravel hidden symmetries, reveal unexpected properties, and provide valuable insights into the realm of shape manipulation.
Our journey begins with a thorough examination of the unique characteristics and geometric properties that define a regular pentagon. We will then delve into the concept of counterclockwise rotation and its profound impact on shapes, setting the stage for our exploration of the specific changes that occur when Pentagon ABCDE undergoes this transformation.
Pentagon Shape

A pentagon is a two-dimensional shape with five sides and five vertices. It is a regular polygon, meaning that all of its sides are equal in length and all of its angles are equal in measure. The interior angles of a regular pentagon measure 108 degrees each.
Geometric Properties
- Five sides of equal length
- Five vertices
- Five interior angles, each measuring 108 degrees
- Two diagonals that intersect at a right angle
- Five-fold rotational symmetry
Rotation Transformation
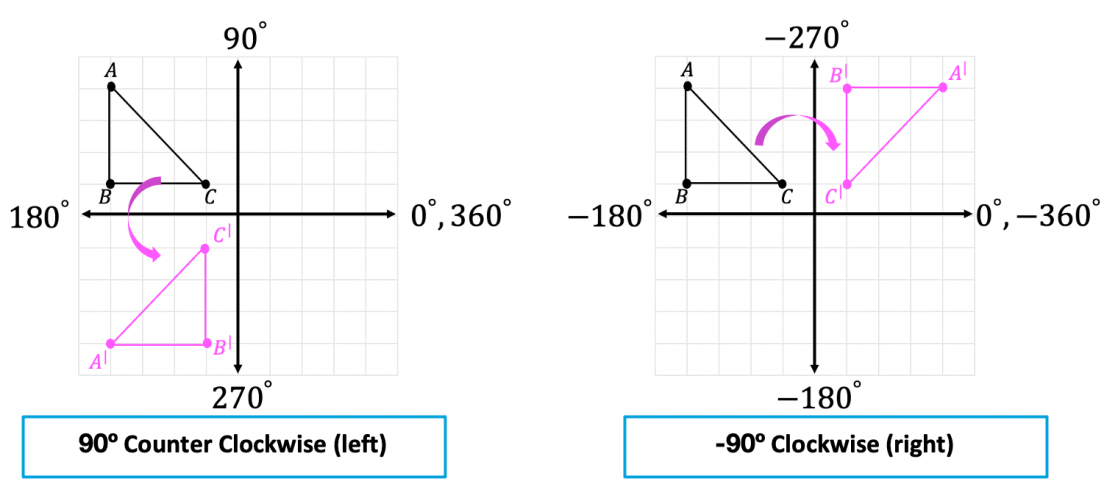
Counterclockwise Rotation
Counterclockwise rotation is a transformation that moves a shape around a fixed point in a circular motion. The direction of the rotation is determined by the right-hand rule, which states that if the thumb of the right hand points in the direction of the rotation, then the fingers will curl in the direction of the rotation.
90-Degree Rotation
A 90-degree rotation is a rotation that moves a shape one-quarter of a full circle. This type of rotation is often used in geometry to study the properties of shapes.
Pentagon Rotation
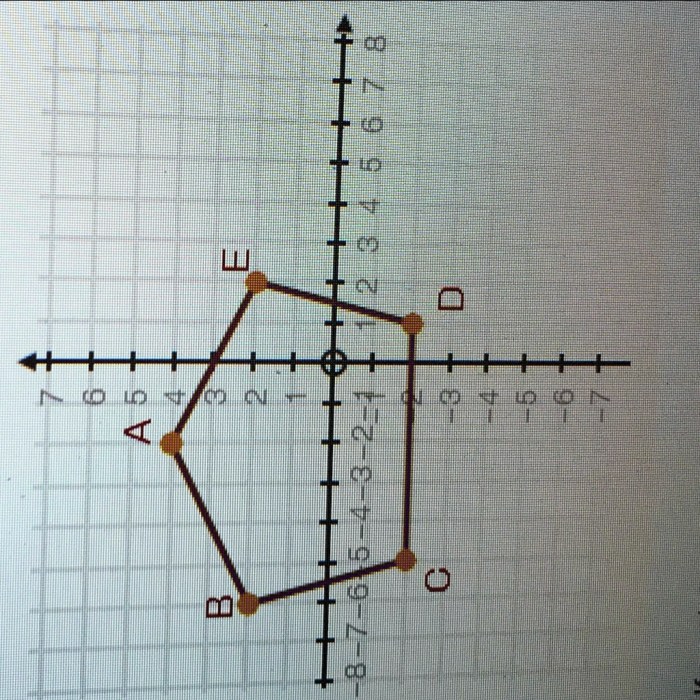
Changes after 90-Degree Counterclockwise Rotation, Pentagon abcde is rotated 90 degrees counterclockwise
- Each side of the pentagon is rotated 90 degrees counterclockwise.
- Each vertex of the pentagon is rotated 90 degrees counterclockwise.
- The interior angles of the pentagon remain the same.
- The diagonals of the pentagon remain perpendicular to each other.
- The five-fold rotational symmetry of the pentagon is preserved.
Visual Representation
[Gambar pentagon yang diputar 90 derajat berlawanan arah jarum jam]
Properties of Rotated Pentagon
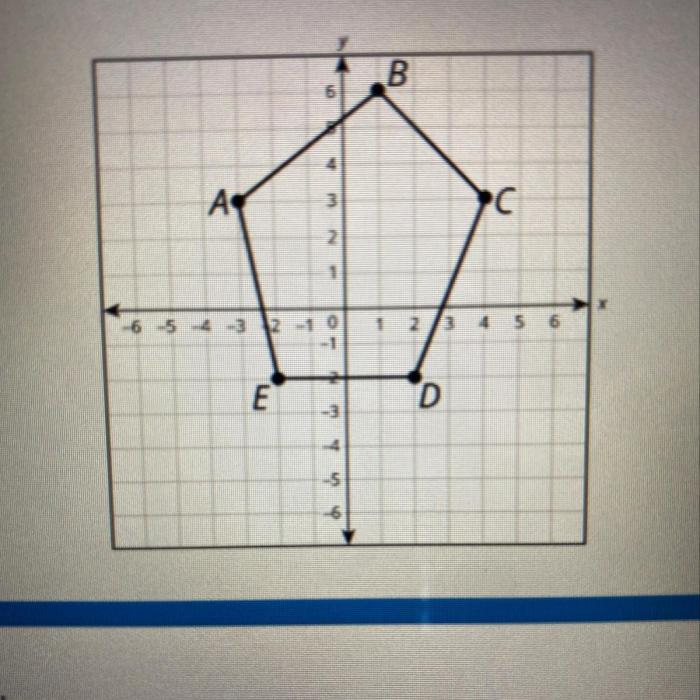
Symmetries
The rotated pentagon has the same symmetries as the original pentagon, including five-fold rotational symmetry and bilateral symmetry.
Geometric Relationships
The diagonals of the rotated pentagon still intersect at a right angle, and the interior angles still measure 108 degrees each.
New Properties
The rotated pentagon may have new symmetries or properties that were not present in the original pentagon. For example, the rotated pentagon may have a higher degree of rotational symmetry or a different type of bilateral symmetry.
Applications and Examples: Pentagon Abcde Is Rotated 90 Degrees Counterclockwise
Understanding the rotation of a pentagon is useful in a variety of applications, including:
Architecture
- Designing buildings with pentagonal shapes
- Creating floor plans that incorporate pentagons
Design
- Creating logos and other visual designs that use pentagons
- Designing fabrics and other materials with pentagonal patterns
Mathematics
- Studying the properties of regular polygons
- Solving geometric problems involving pentagons
Helpful Answers
What is the effect of rotating a pentagon 90 degrees counterclockwise?
Rotating a pentagon 90 degrees counterclockwise results in a new pentagon that is shifted by a quarter of its perimeter and exhibits different orientations of its vertices and sides.
What symmetries remain unchanged after rotating a pentagon 90 degrees counterclockwise?
The rotational symmetry of order 5 and the reflectional symmetries along the diagonals remain unchanged after the rotation.
What new properties emerge after rotating a pentagon 90 degrees counterclockwise?
The rotated pentagon may exhibit new symmetries, such as reflectional symmetry along the axes that pass through the midpoints of opposite sides.